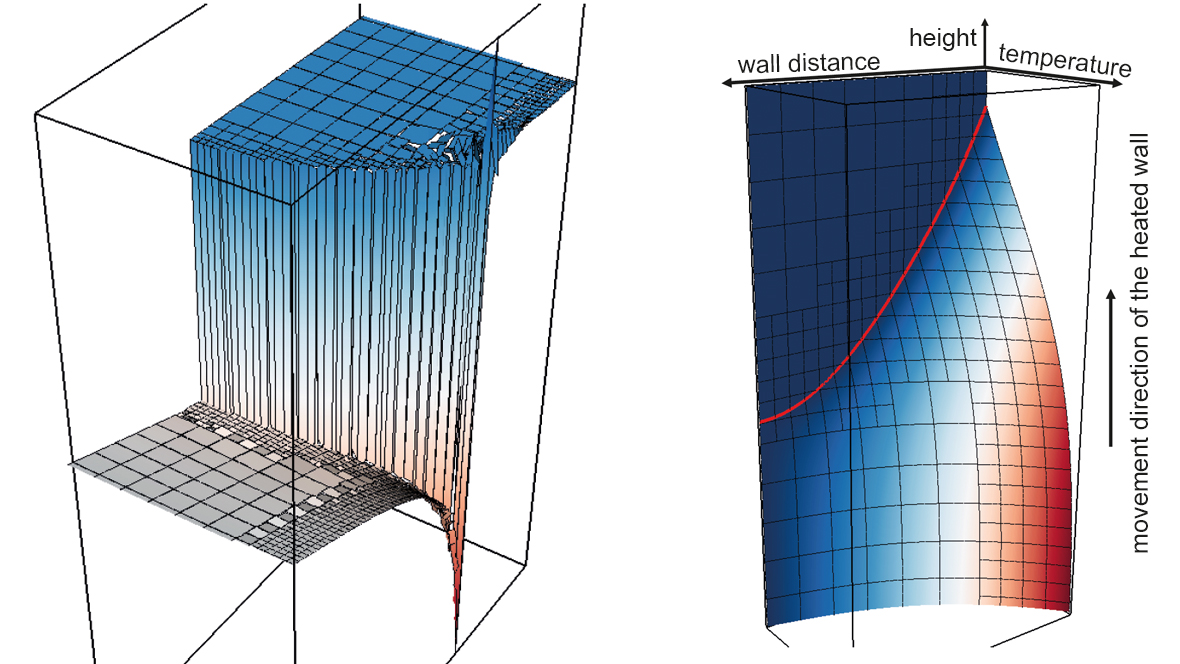
Overview
One focus of B06 is the application of the numerical schemes from the first funding period to experiments of the CRC, especially those with phase transitions. By connecting the inhouse Extended Discontinuous Galerkin (XDG) code (see also Kummer 2016 and Kummer et al. 2017) to the OpenFOAM infrastructure, the DG method can be applied within other CRC projects, e.g. to phase field models in cooperation with B07. The second focus is the continuation of contact line modelling with the XDG method. Due to its high local accuracy, this method is particularly suitable for the investigation of thermodynamic inconsistencies of contact line models. Moreover, singularities of the flow fields can be treated by means of local basis functions.
Team
| Name | Contact | |
|---|---|---|

| Dr.-Ing. Florian Kummer | kummer@fdy.tu-... +49 6151 16-26194 L1|01 324 |

| Matthias Rieckmann M.Sc. | rieckmann@fdy.tu-... L1|01 325 |
Associated members
| Name | Contact | |
|---|---|---|
|
| Prof. Dr.-Ing Martin Oberlack | office@fdy.tu-... +49 6151 16-26200 L1|01 332 |

| Dr.-Ing. Martin Smuda | smuda@fdy.tu-... +49 6151 16-26196 L1|01 325 |
Publications and conference contributions

Error on loading data
An error has occured when loading publications data from TUbiblio. Please try again later.
-
{{ year }}
-
; {{ creator.name.family }}, {{ creator.name.given }}{{ publication.title }}.
; {{ editor.name.family }}, {{ editor.name.given }} (eds.); ; {{ creator }} (Corporate Creator) ({{ publication.date.toString().substring(0,4) }}):
In: {{ publication.series }}, {{ publication.volume }}, In: {{ publication.book_title }}, In: {{ publication.publication }}, {{ publication.journal_volume}} ({{ publication.number }}), ppp. {{ publication.pagerange }}, {{ publication.place_of_pub }}, {{ publication.publisher }}, {{ publication.institution }}, {{ publication.event_title }}, {{ publication.event_location }}, {{ publication.event_dates }}, ISSN {{ publication.issn }}, e-ISSN {{ publication.eissn }}, ISBN {{ publication.isbn }}, DOI: {{ publication.doi.toString().replace('http://','').replace('https://','').replace('dx.doi.org/','').replace('doi.org/','').replace('doi.org','').replace("DOI: ", "").replace("doi:", "") }}, Official URL, {{ labels[publication.type]?labels[publication.type]:publication.type }}, {{ labels[publication.pub_sequence] }}, {{ labels[publication.doc_status] }} - […]
-
Number of items in this list: >{{ publicationsList.length }}
Only the {{publicationsList.length}} latest publications are displayed here.